Understanding the Relationship between Voltage and Electric Field: A Journey Through Potential
Imagine a world where you can sense the invisible forces around you, forces that pull and push, shaping the behavior of charged objects. This is the world of electric fields, a realm where voltage plays a crucial role.
Voltage: The Key to Electric Potential
Voltage is a measure of electrical potential energy per unit charge. It represents the difference in electrical potential between two points in an electric field. Think of it like a difference in height between two points on a hill. The higher the point, the greater its potential energy. Similarly, a higher voltage indicates a greater potential energy for a charged particle.
Voltage and Electric Potential Energy
The relationship between voltage and electric potential energy is straightforward. The voltage between two points is the change in potential energy per unit charge. In other words:
Voltage (V) = Potential Energy Difference (ΔU) / Charge (Q)
This means that a higher voltage implies a greater change in potential energy for a charged particle moving between those points.
Electric Field: The Force Behind Voltage
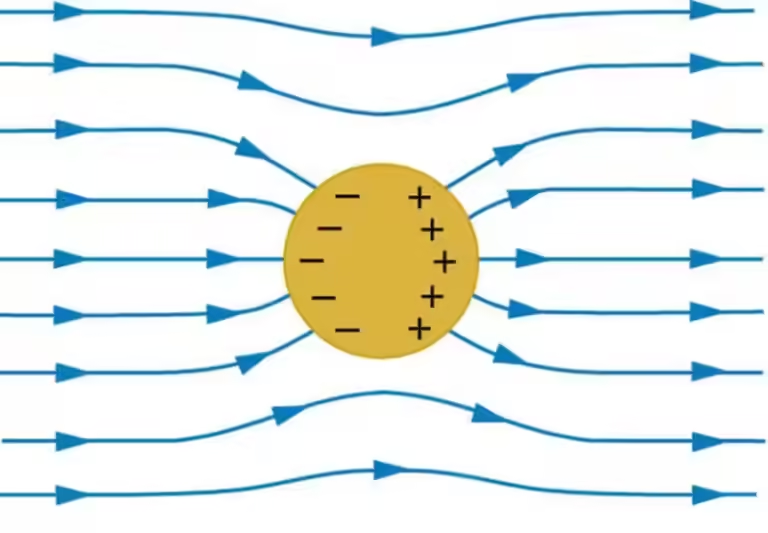
The electric field is a region of space where a charged object experiences a force. It's a vector quantity, meaning it has both magnitude and direction. The strength of the electric field at a particular point is defined as the force per unit charge that a test charge would experience at that point.
Electric Field Strength and Force
The force experienced by a charge (q) in an electric field (E) is given by:
Force (F) = Charge (q) Electric Field (E)
This equation shows that the force on a charged particle in an electric field is proportional to both the charge and the electric field strength.
The Connection: Voltage and Electric Field
The electric field and voltage are inextricably linked. The relationship between them can be described as follows:
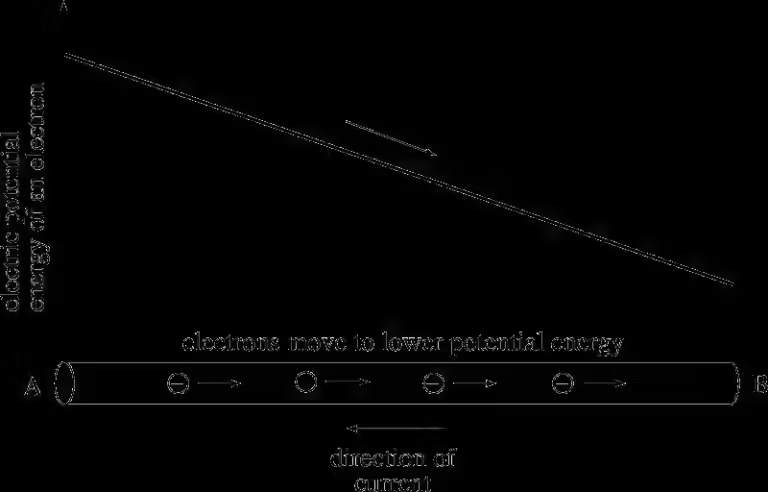
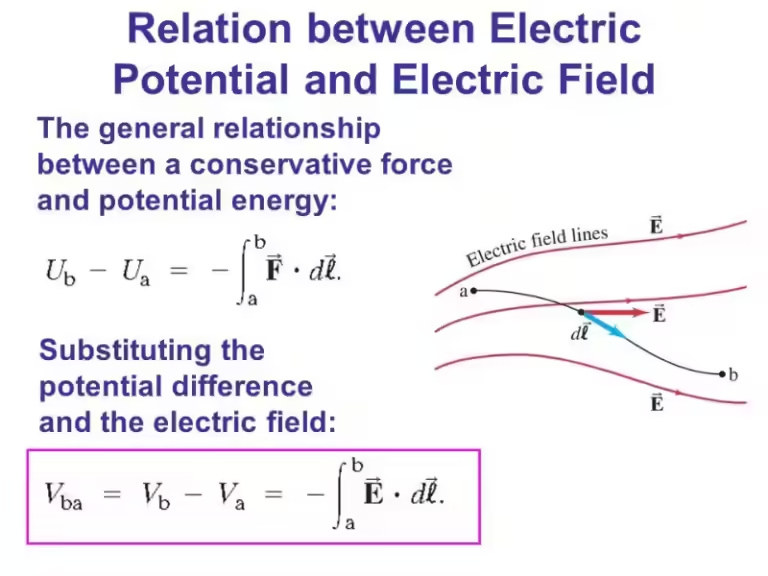
- The electric field is the negative gradient of the voltage. This means that the electric field at a point is the rate of change of voltage with respect to distance.
- A strong electric field corresponds to a rapid change in voltage. This is analogous to a steep slope on a hill, where the potential energy changes rapidly over a short distance.
Mathematical Representation
Mathematically, the relationship between the electric field (E) and the voltage (V) can be expressed as:
Electric field (E) = - Gradient of Voltage (V)
This equation is fundamental to understanding how electric fields arise from voltage differences.
Visualizing the Relationship: A Topographical Analogy
To visualize the relationship between voltage and electric field, we can use the analogy of a topographical map. Imagine a map with contour lines representing lines of equal elevation. The spacing between these contour lines indicates the steepness of the terrain.
- The voltage is analogous to elevation. The higher the elevation, the greater the potential energy.
- The electric field is analogous to the slope of the terrain. The closer the contour lines, the steeper the slope and the stronger the electric field.
The Power of Visualization
This analogy allows us to visualize the electric field as a force that pushes charged particles along paths of steepest descent, analogous to a ball rolling down a hill. The voltage difference between two points represents the potential energy difference that drives this motion.
Applications and Implications
The relationship between voltage and the electric field is fundamental to understanding various electrical phenomena, including:
- Capacitors: Capacitors store electrical energy by creating an electric field between two conductive plates. The voltage across the capacitor determines the strength of this electric field.
- Circuits: In electrical circuits, voltage differences drive the flow of current, which is the movement of charged particles through conductors. The electric field created by the voltage difference propels these charges.
- Electrostatic forces: The electric field surrounding charged objects is responsible for the electrostatic forces of attraction and repulsion between them.
The relationship between voltage and the electric field provides a powerful lens through which to understand the forces that govern the behavior of charged objects. By understanding the concept of potential energy and its relationship to electric fields, we can gain a deeper appreciation for the invisible forces that shape our world.
Frequently Asked Questions about Voltage and Electric Field
What is the relationship between voltage and electric field?
The electric field is the negative gradient of the voltage. In simpler words, the electric field at any point is the rate of change of voltage with respect to distance in that direction.
How can I visualize the relationship between voltage and electric field?
Imagine a topographical map where contour lines represent equipotential surfaces (lines of constant voltage). The closer the contour lines, the stronger the electric field. The electric field is always perpendicular to these equipotential surfaces.
What are the units for electric field and voltage?
Electric field is measured in units of Newtons per Coulomb (N/C) or Volts per meter (V/m). Voltage is measured in Volts (V).
Can I use voltage to find the electric field created by a charge distribution?
Yes, you can calculate the electric field by taking the negative gradient of the voltage. This can be much easier than directly calculating the electric field from the charge distribution, especially for complex geometries.
What is the significance of the negative sign in the relationship between electric field and voltage?
The negative sign indicates that the electric field points in the direction of decreasing voltage. In other words, a positive charge will move from a region of higher voltage to a region of lower voltage.
How is the relationship between voltage and electric field important in electrostatics?
This relationship is fundamental in electrostatics. It allows us to analyze the behavior of capacitors, understand electric fields around conductors, and design electronic circuits.